A hypocycloid is generated by the tracing a fixed point on a circle in which that circle rolls along the inside edge of the larger circle. I took some time to create a few of my own hypocycloid's using a GeoGebra software. My artistic side kind of got the better of me when doing this exercise as the numerous shapes and designs that could be created were intriguing.
Cardano, a man of many interests of the 16th century, studied hypocycloid's which later became known as Cardano circles. The construction of high speed printing presses were based off of these circular patterns. It would be interesting to see how hypocycloid's and even cycloid's are incorporated in to everyday life. I am sure the engineering world has a lot of use for these designs, but would rather see them as a mechanical curve instead of just a geometrical circle.
Link to Geogebra tool i used: http://www.geogebratube.org/material/show/id/29389
References:
http://math27.page.tl/Gerolamo-Cardano.htm
http://library.iated.org/view/FLOREZ2011DES

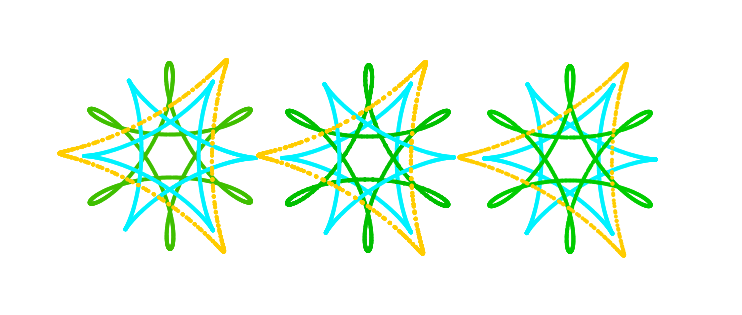
Clarify the difference: epicycloid, hypocycloid.
ReplyDeleteYou could make this an exemplar by sharing your design thinking, or sharing the mathematical properties of the circles (the ratio is important) that created the designs you like.
Do like your designs, though! Oh - you should link to the GGB tool you used. (Even if it was mine. And if it wasn't - I definitely want to know.)
Very interesting! I had no idea what a hypocycloid was and I thought you did a great job creating one in geogebra, I'm sure it wasn't easy. I am not create at all so there's no way I could have made one myself.
ReplyDelete